James Madison University - Department of Geology & Environmental Science
Lynn S. Fichter
Steve Baedke
Eric Pyle
Steve WhitmeyerTeaching Chaos and Complex Evolutionary Systems Theories at the Introductory Level
Model: Strange Attractors in Phase Space
Strategies and Rubrics for Teaching Chaos and Complex Systems Theories as Elaborating, Self-Organizing, and Fractionating Evolutionary Systems,
Fichter, Lynn S., Pyle, E.J., and Whitmeyer, S.J., 2010, Journal of Geoscience Education (in press)Description: In mathematics an attractor is a region of phase space that "attracts" all nearby points as time passes. A phase space is simply an XY or XYZ graph (although it could be at higher dimensions) with variables such as position and velocity. The changing values of the system have a trajectory which moves across the phase space through time toward the attractor, like a ball rolling down a hilly landscape toward the valley it is attracted to. Each dynamical system and each ‘r’ value generates a particular type of attractor.
There are three basic kinds of attractors. A point attractor is generated when a low energy system decays to equilibrium, like the diminishing swings of a pendulum to motionlessness. In phase space this appears as a single point (or a spiral down to a single point). In the logistic system time series it appears as the population attenuating to one value (Figure 1).
A limit cycle attractor repeats the exact same trajectory in phase space, like a clock pendulum driven by some constant energy source (although in fact clock pendulums always exhibit some strange attractor irregularity, sometimes called pink or 1/f noise.) In the logistic system the limit cycle attractor is an exact oscillation among two or more population sizes, out to the 7 decimal places the program shows (Figure 2) (e.g. ‘r’ value = 3.1).
A strange attractor is one which in phase space has a recognizable shape, but never exactly repeats its trajectory. The Lorenz attractor is the best known (an internet search for images produces thousands of examples; e.g. example, example, example.) Strange attractors are the iconic behavior of chaos/complex systems.
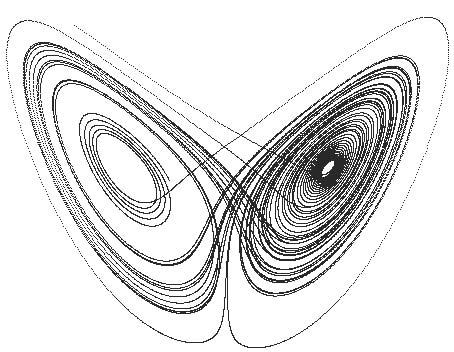
In a classical framework—a system that descends directly to equilibrium—the only attractor is the point; such systems are non-evolutionary, and we are not interested in them in this discourse. Of course, we model some systems as limit cycles, such as a driven pendulum, but that can only be sustained by the continuous input of moderate amounts of energy. These are also non-evolutionary but we are moderately interested in these because, with just a little more energy, they will transform into evolutionary strange attractors that passes through a series of bifurcations as energy increases. But, as we see with the logistic system, if the energy dissipation level continues to rise, the system undergoes a series of accelerating bifurcations until we see a non-repeating pattern, which plots in phase space as a strange attractor. From an evolutionary viewpoint, only the strange attractor is dissipating enough energy—is unstable enough—to be able shift—evolve—into an alternative pattern.
Presentation: We use a Lorenz computer program (might need this .dll file) that shows evolution of the Lorenz strange attractor, although Cross (2009) has a nice applet of the system with suggestions for experimenting with the system on line.
Anticipated Learning Outcome:
- 14. Complex systems have behaviors that may superficially appear random, but have recognizable larger scale patterns. The weather is an example. Forecasters have trouble predicting the exact weather 3 days out, but the basic pattern of moving high and low pressure systems is universal. Likewise, this spring may not have been like the last spring, but the seasons follow in the same order every time.