Teaching Chaos and Complex Evolutionary Systems Theories at the Introductory Level

(Back to Self Similarity)    (To Feigenbaum Ratio)

Model: Fractals:
More Depth on Self Similarity

Strategies and Rubrics for Teaching Chaos and Complex Systems Theories as Elaborating, Self-Organizing, and Fractionating Evolutionary Systems,

Fichter, Lynn S., Pyle, E.J., and Whitmeyer, S.J., 2010, Journal of Geoscience Education (in press)
Description: In some classes we need a deeper understanding of fractals. Their close affinity with power-laws is important in understanding complex systems, and the behavior of natural systems. Our goal in introductory classes is not to present a treatise on fractal geometry, because it would be possible to spend hours of class time just getting started, and fractals are so fascinating, so ubiquitous, and so important that it is tempting to spend more and more time exploring them. Mainly what we want is for students to realize there is another geometry besides Euclidean, and that this geometry is how the natural world is constructed.
      What students need to understand are the two signature properties of fractals; (1) fractal objects are generated by the iteration of a simple algorithm, that is, they develop by an evolutionary process, usually elaboration and self-organization. (which harkens them back to the logistic time series, which is generated by iteration), and (2) fractal objects have non-whole number dimensions. We can introduce both of these ideas in about 15 minutes, enough to build on later if we need to. Showing a variety of examples to show fractal ubiquitousness is, at this stage, more important than the mathematics.
      There are innumerable books and web sites that explore fractals, from basic to high mathematical sophistication. One very accessible book to the mathematics is Liebovitch (1998,Fractals and Chaos Simplified for the Life Sciences), while Briggs (1992, Fractals: The Patterns of Chaos: Discovering a New Aesthetic of Art, Science, and Nature) explores and illustrates the many ways fractals show up in nature and art. An Amazon search under Books/Fractals had over 2500 hits.

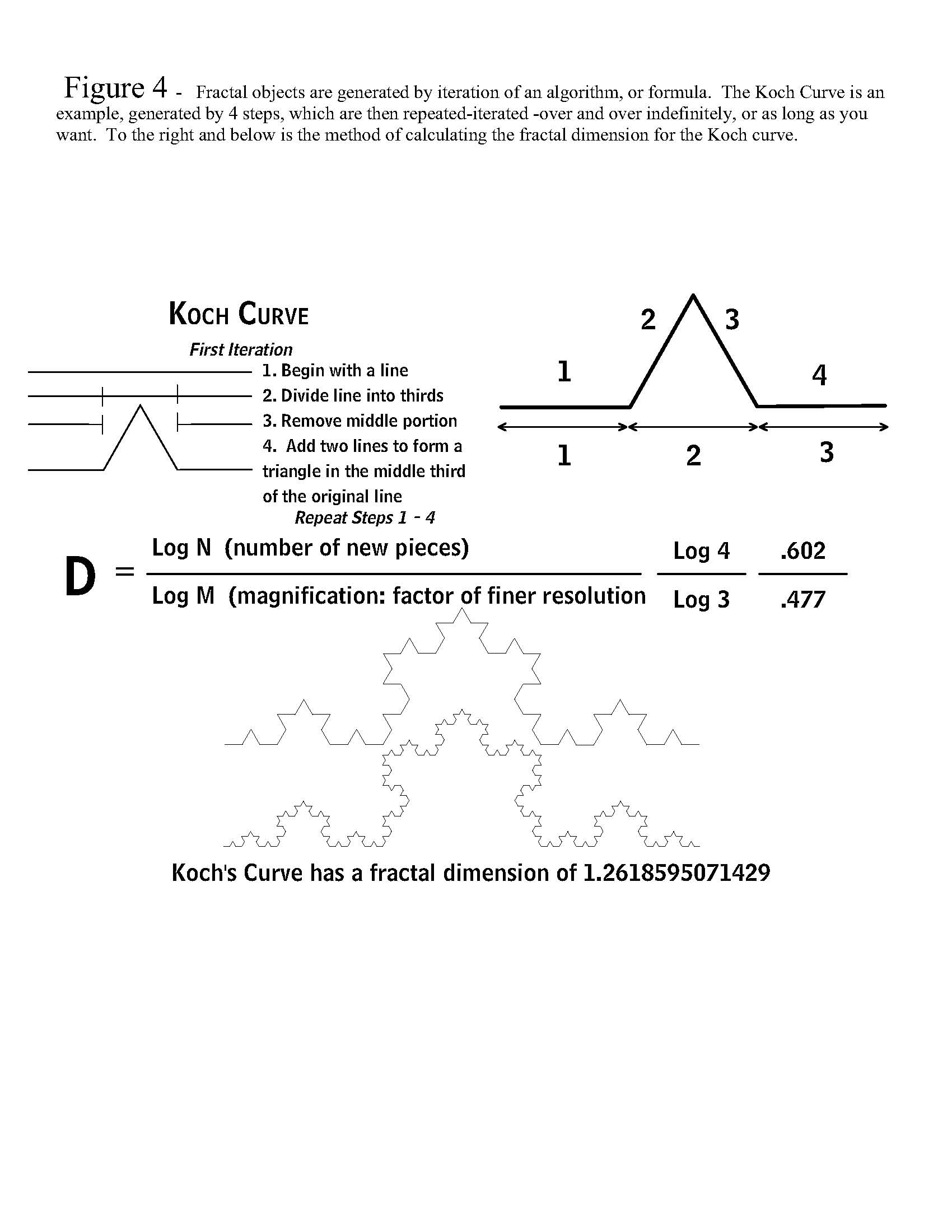
Presentation: Fractals are generated by an iterative process and we use the iconic Koch curve (or snowflake) as our class room demonstration (Figure 4). We have an animated Fractal Geometry Power Point that illustrates this in less than 5 minutes. The algorithm is: 1) begin with a line, 2) divide the line into thirds, 3) remove the middle third, 4) fill the middle third space with two lines the length of the thirds, forming a triangle, repeat on each of the new lines. The Koch curve is so common that a search for “Koch curve’ through Google/Images results in thousands of hits.

     Fractals also have non-whole number dimensions. In mathematics the dimension of an object is calculated by D = Log N/ Log M, where N is the number of new pieces generated by an iteration (for the Koch curve from the initial 1 to 4), and M is the magnification that would be necessary to take each new piece generated by the iteration and enlarge it to the size of the original (for the Koch curve this is 3). Thus, the dimension of the Koch curve is D = Log 4/Log 3 or .602/.477 which gives a fractal dimension of 1.26185. . . In some classes we use the same mathematics to demonstrate why one, two, and three dimensional Euclidean objects have those whole number dimensions.

      There is so much more that can be done with fractals and their applications, and we sometimes do additional things, but at the introductory level this is where we stop.

Anticipated Learning Outcome:
    8. There is no typical or average size of events or objects; they come nested inside each other, patterns within patterns, within patterns. Peripheral to these discussions, but crucial when dealing with statistics, is that normal statistical analysis cannot be used on fractally organized data sets; there is no mean or standard deviation for fractal objects.
    9. Unlike the geometry we are usually taught, most natural objects have non-whole number dimensions.

Figure 4 - Generating a fractal object by iteration, and fractal dimensions.



Fractal Geometry Power Point


(Back to Self Similarity)    (To Feigenbaum Ratio)