(Back to Fractals)    (To Increasing Instability)

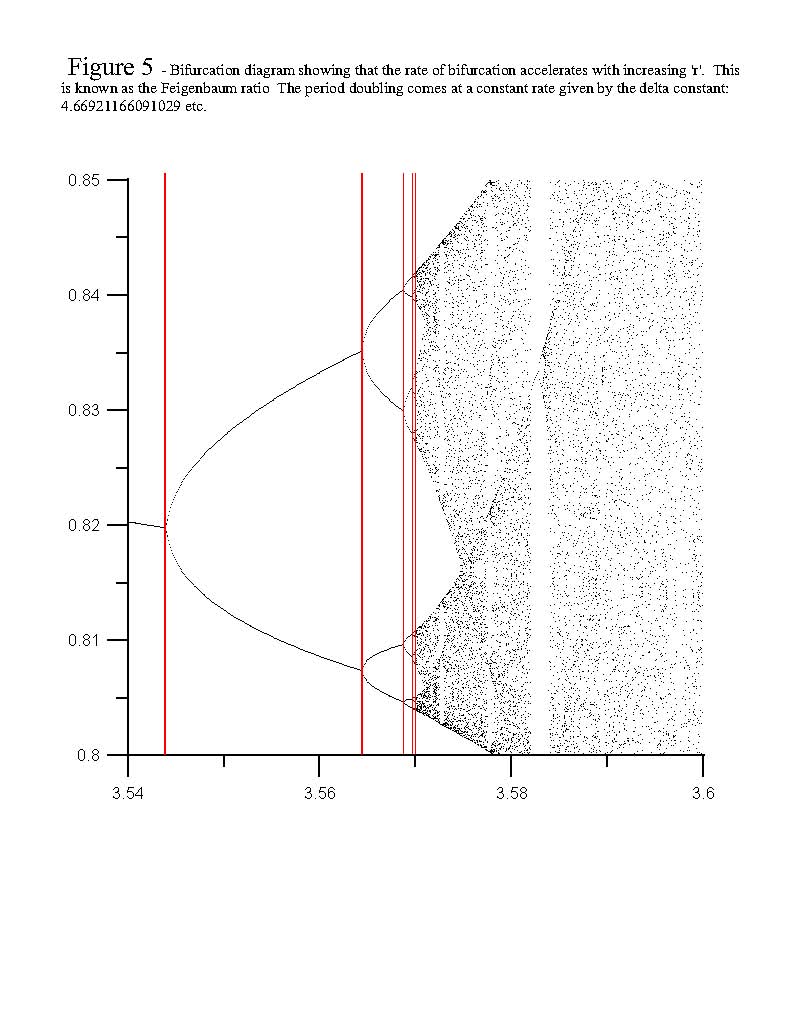
Model: Feigenbaum Ratio: Change Comes Faster at a Constant Ratio

Bifurcation Diagram - Part 3 of 4 Parts

Strategies and Rubrics for Teaching Chaos and Complex Systems Theories as Elaborating, Self-Organizing, and Fractionating Evolutionary Systems,

Fichter, Lynn S., Pyle, E.J., and Whitmeyer, S.J., 2010, Journal of Geoscience Education (in press)

---

Description: Mathematically, Feigenbaum demonstrated that period doubling comes at a constant rate given by the delta constant: 4.66921166091029..., and that this constant arises in any dynamical system that approaches chaotic behavior via period-doubling bifurcations: fluid-flow turbulence, electronic oscillators, chemical reactions, and even the Mandelbrot set.

Presentation: In introductory presentations we do not go into the mathematics of this, but while we have a bifurcation diagram up it is easy to demonstrate that each bifurcation comes faster than the previous one. In Power Point we just draw a series of vertical lines at each bifurcation and note that they come faster and faster (Figure 5).

Anticipated Learning Outcome:

10. All complex systems accelerate their rate of change—bifurcations—at the same rate. This is a universality property; if this rate of change in behavior of a system is detected, the system is a complex system.

---

Power Point

Figure 5 - Zooming in on the Bifurcation Diagram

(Back to Fractals)    (To Increasing Instability)

---